一般化した平均であるヘルダー平均の性質を紹介します。
ヘルダー平均の性質(指数の関係)
\(-\infty\leq p\leq q\leq \infty\) を満たす \(p, q\) に対して \[M_p(x_1,x_2, \ldots,x_n)\leq M_q(x_1,x_2, \ldots,x_n)\] また \(p<q\) のとき, \[M_p(x_1,x_2, \ldots,x_n)=M_q(x_1,x_2, \ldots,x_n)\Longleftrightarrow x_1=x_2=\cdots=x_n\]
証明
証明の方針として
- 正の実数 \(p\) に対して \(M_{-p}(x_1,x_2, \ldots,x_n)\leq M_0(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\)
- \(0< p\leq q\) に対して \(M_p(x_1,x_2, \ldots,x_n)\leq M_q(x_1,x_2, \ldots,x_n)\)
- \(q\leq p<0\) に対して \(M_q(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\)
- \(0\) ではない実数 \(p\) に対して \(M_{-\infty}(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\leq M_\infty(x_1,x_2, \ldots,x_n)\)
を示す.
1.の証明
まず 正の実数 \(p\) に対して \[M_0(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\] を示す.
\[M_{p}(x_1,x_2, \ldots,x_n)=\left(\frac{1}{n}\sum_{i=1}^nx_i^p\right)^\frac{1}{p},\ M_0(x_1,x_2, \ldots,x_n)=\left(\prod_{i=1}^nx_i\right)^\frac{1}{n}\]
であるから
\[\begin{eqnarray*}
&\left(\frac{1}{n}\sum_{i=1}^nx_i^p\right)^\frac{1}{p}&\geq&\left(\prod_{i=1}^nx_i\right)^\frac{1}{n}\\
\Longleftrightarrow& \frac{1}{p}\ln\left(\frac{1}{n}\sum_{i=1}^nx_i^p\right)&\geq&\frac{1}{n}\sum_{i=1}^n\ln x_i\\
\Longleftrightarrow& \ln\left(\frac{1}{n}\sum_{i=1}^nx_i^p\right)&\geq&\frac{1}{n}\sum_{i=1}^n\ln x_i^p
\end{eqnarray*}\]
を示せばよいが, 最後の不等式は \(\ln x\) \(x>0\) で凹関数(concave function)なので, イェンゼンの不等式(Jensen’s inequality)より従う. ゆえに \(M_0(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\) である.
さらに, 等号が成立するのは \(x_1=x_2=\cdots=x_n\) の時のみであることもイェンゼンの不等式から従う.
また, \[M_{-p}(x_1,x_2, \ldots,x_n)=\left(\frac{1}{n}\sum_{i=1}^nx_i^{-p}\right)^{-\frac{1}{p}}=\frac{1}{\left(\displaystyle\frac{1}{n} \sum_{i=1}^n\left(\frac{1}{x_i}\right)^p\right)^\frac{1}{p}}=\frac{1}{M_p(\frac{1}{x_1},\frac{1}{x_2}, \ldots,\frac{1}{x_n})}\] であり, 上より
\[M_p\left(\frac{1}{x_1},\frac{1}{x_2}, \ldots,\frac{1}{x_n}\right)\geq M_0\left(\frac{1}{x_1},\frac{1}{x_2}, \ldots,\frac{1}{x_n}\right)=\sqrt[n]{\frac{1}{x_1x_2\cdots x_n}}=\frac{1}{M_0(x_1,x_2, \ldots,x_n)}\] なので, \(M_{-p}(x_1,x_2, \ldots,x_n)\leq M_0(x_1,x_2, \ldots,x_n)\) が従う.
等号の成立は \(x_1=x_2=\cdots=x_n\) の時のみ.
以上より, 正の実数 \(p\) に対して \[M_{-p}(x_1,x_2, \ldots,x_n)\leq M_0(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\]
2.の証明
\(0< p\leq q\) の時, \(f(x)=x^{\frac{p}{q}}\) は \(x>0\) で凹関数である. よってイェンゼンの不等式より \[\frac{1}{n}\sum_{i=1}^n x_i^p=\frac{1}{n}\sum_{i=1}^n x_i^{q\cdot\frac{p}{q}}\leq\left(\frac{1}{n}\sum_{i=1}^nx_i^q\right)^{\frac{p}{q}}\] 両辺の \(p\) 乗根を考えれば \[\left(\frac{1}{n}\sum_{i=1}^nx_i^p\right)^{\frac{1}{p}}\leq\left(\frac{1}{n}\sum_{i=1}^nx_i^q\right)^{\frac{1}{q}}\] ゆえに \[M_p(x_1,x_2, \ldots,x_n)\leq M_q(x_1,x_2, \ldots,x_n)\] \(p<q\) のとき, 等号成立は \(x_1=x_2=\cdots=x_n\) の時のみ.
3.の証明
\(q\leq p<0\) の時, \(0<-p\leq-q\) なので
\[\begin{eqnarray*}
M_p(x_1,x_2, \ldots,x_n)&=&\frac{1}{M_{-p}\left(\frac{1}{x_1},\frac{1}{x_2}, \ldots,\frac{1}{x_n}\right)}\\
M_q(x_1,x_2, \ldots,x_n)&=&\frac{1}{M_{-q}\left(\frac{1}{x_1},\frac{1}{x_2}, \ldots,\frac{1}{x_n}\right)}\\
M_{-q}\left(\frac{1}{x_1},\frac{1}{x_2}, \ldots,\frac{1}{x_n}\right)&\geq&M_{-p}\left(\frac{1}{x_1},\frac{1}{x_2}, \ldots,\frac{1}{x_n}\right)
\end{eqnarray*}\]
ゆえに \[M_q(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\] \(q<p\) のとき, 等号成立は \(x_1=x_2=\cdots=x_n\) の時のみ.
4.の証明
\(x_1\geq x_2\geq\cdots\geq x_n\) としても一般性を失わない. このとき
\[M_{p}(x_1,x_2, \ldots,x_n)=\left(\frac{1}{n}\sum_{i=1}^nx_i^p\right)^\frac{1}{p}\leq \left(\frac{1}{n}\sum_{i=1}^nx_1^p\right)^\frac{1}{p}=x_1\] であり,等号成立は \(x_1=x_2=\cdots=x_n\) の時.
\[M_{p}(x_1,x_2, \ldots,x_n)=\left(\frac{1}{n}\sum_{i=1}^nx_i^p\right)^\frac{1}{p}\geq \left(\frac{1}{n}\sum_{i=1}^nx_n^p\right)^\frac{1}{p}=x_n\] であり, 等号成立は \(x_1=x_2=\cdots=x_n\) の時. よって \[M_{-\infty}(x_1,x_2, \ldots,x_n)\leq M_p(x_1,x_2, \ldots,x_n)\leq M_\infty(x_1,x_2, \ldots,x_n)\] が従う.
以上より, 1~4を合わせると
\(-\infty\leq p\leq q\leq \infty\) を満たす \(p, q\) に対して \[M_p(x_1,x_2, \ldots,x_n)\leq M_q(x_1,x_2, \ldots,x_n)\] となる.
また, \[M_0(x_1,x_2, \ldots,x_n)=M_\infty(x_1,x_2, \ldots,x_n)\\M_0(x_1,x_2, \ldots,x_n)=M_{-\infty}(x_1,x_2, \ldots,x_n)\\M_\infty(x_1,x_2, \ldots,x_n)=M_{-\infty}(x_1,x_2, \ldots,x_n)\] がそれぞれ成立するのは \(x_1=x_2=\cdots=x_n\) の時なので, \(p<q\) のとき, \[M_p(x_1,x_2, \ldots,x_n)=M_q(x_1,x_2, \ldots,x_n)\Longleftrightarrow x_1=x_2=\cdots=x_n\] も分かる.
この性質から \[\min\{x_1,x_2, \ldots,x_n\}\leq M_p(x_1,x_2, \ldots,x_n)\leq\max\{x_1,x_2, \ldots,x_n\}\]や、相加平均・相乗平均・調和平均の関係\[ M_{-1}(x_1,x_2, \ldots,x_n)\leq M_0(x_1,x_2, \ldots,x_n)\leq M_1(x_1,x_2, \ldots,x_n)\]が分かります。
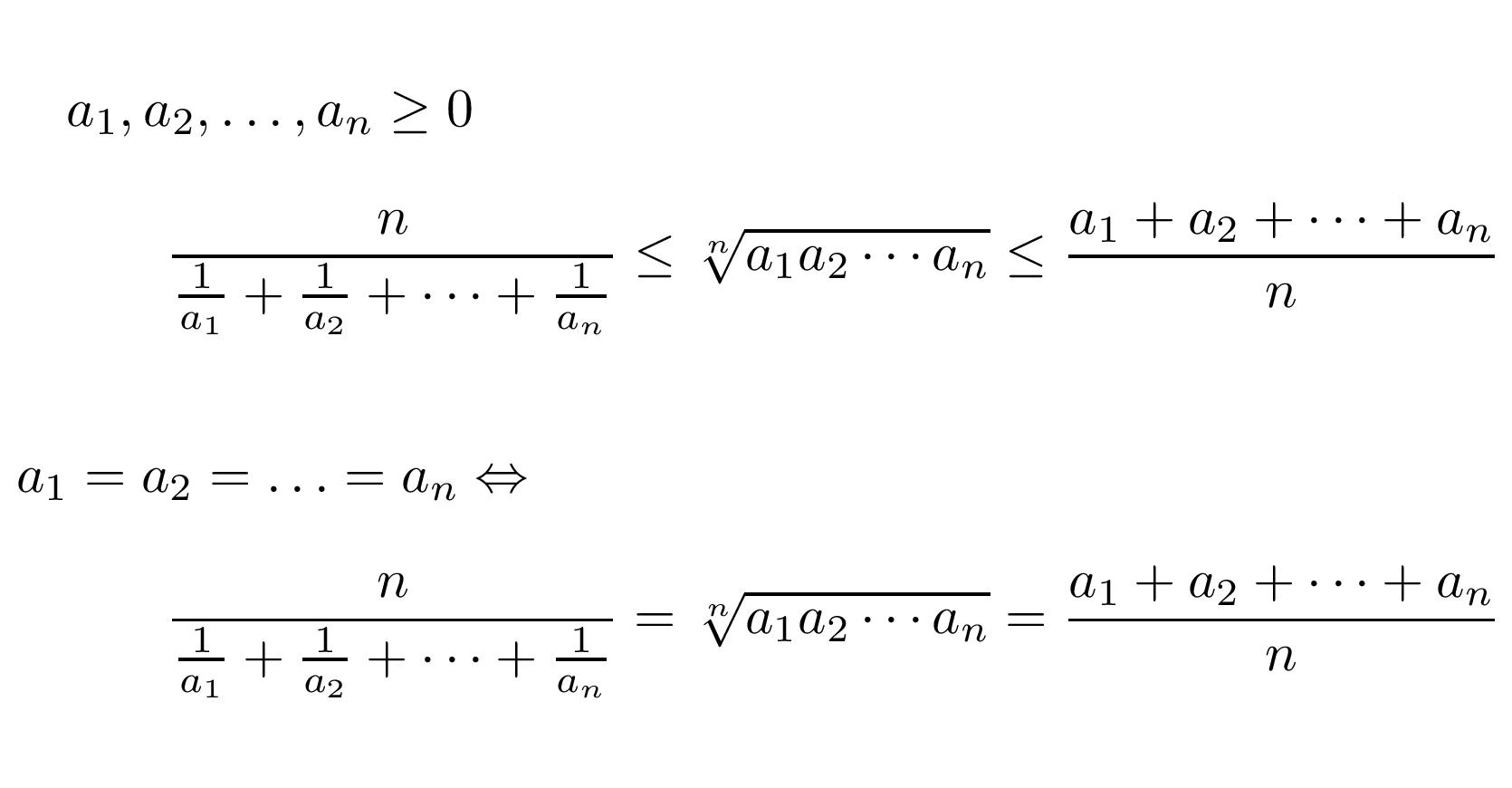

コメント