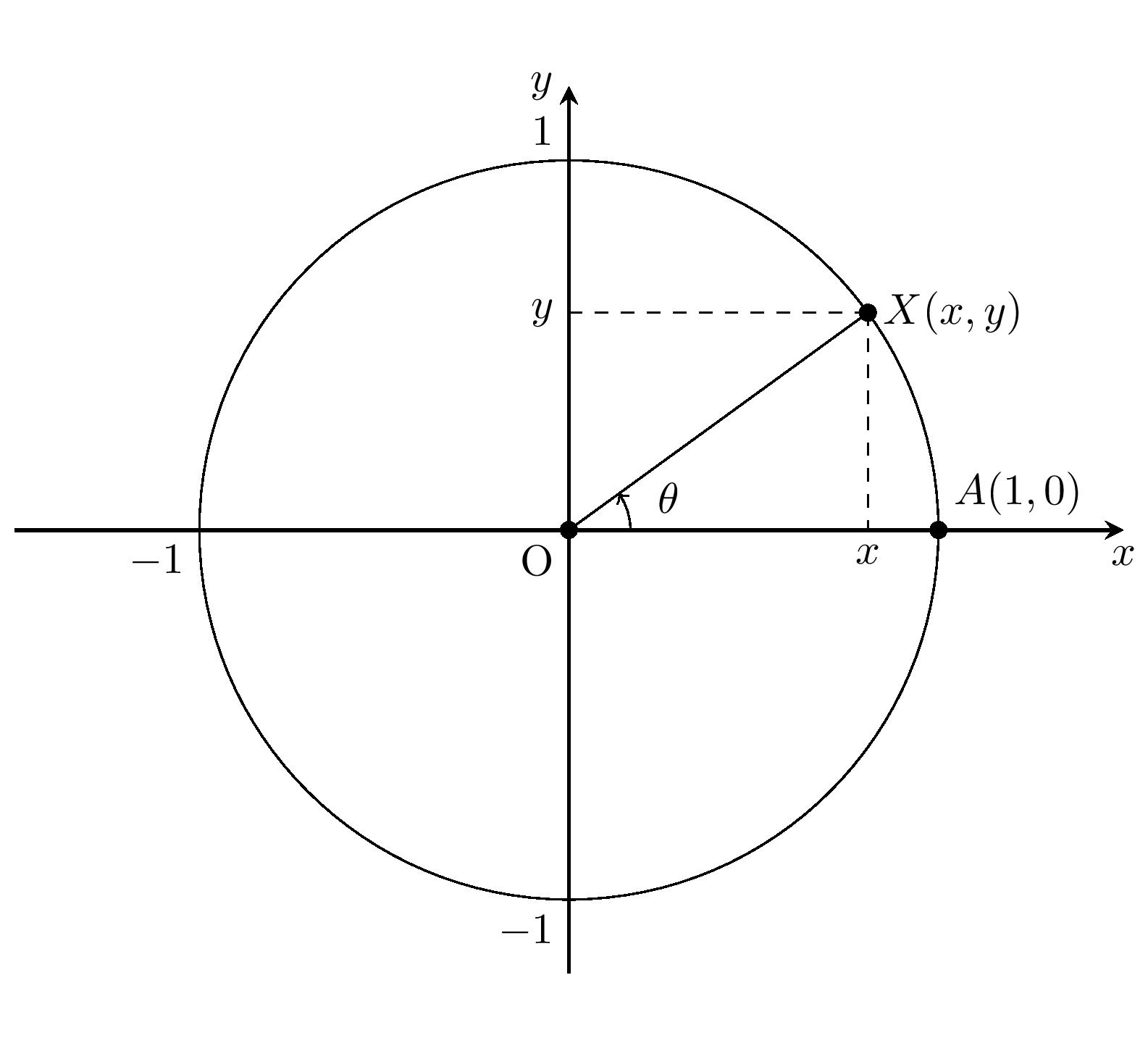
三角関数の定義は様々なものがあるが、ここでは単位円と角度を用いた定義を紹介する.
座標平面(\(\mathbf{R}^2\))を考える. 原点\(O(0, 0)\), 点\(A(1, 0)\)として, 点\(X(x, y)\)は単位円 \(x^2+y^2=1\) 上を動くものとする.
動径 \(OX\) が始線 \(OA\) とのなす角の1つを \(\theta\) と置く. この時,

\[ \begin{eqnarray*}\sin \theta &=& y \\ \cos \theta &=& x \\ \tan \theta &=& \frac{y}{x}=\frac{\sin \theta}{\cos \theta}\end{eqnarray*}\]
で \(\sin, \cos, \tan\) を定義する. \(\sin\) を正弦, \(\cos\) を余弦, \(\tan\) を正接と呼ぶ.
性質
定義から以下の事が分かる.
基本相互関係
\[ \sin^2\theta + \cos^2 \theta = 1 \\ 1 + \tan^2 \theta = \frac{1}{\cos^2 \theta}\]
周期
\[\begin{eqnarray*}\sin(\theta+2\pi n)&=&\sin\theta \\ \cos(\theta+2\pi n)&= &\cos\theta\\ \tan(\theta+2\pi n)&= &\tan\theta\end{eqnarray*}\]
負角
\[\begin{eqnarray*}\sin(-\theta)&=&-\sin\theta \\ \cos(-\theta)&=&\cos\theta \\ \tan(-\theta)&=&-\tan \theta \end{eqnarray*}\]
余角
\[\begin{eqnarray*}\sin(\frac{\pi}{2}-\theta)&=&\cos\theta \\ \cos(\frac{\pi}{2}-\theta)&=&\sin\theta \\ \tan(\frac{\pi}{2}-\theta)&=&\frac{1}{\tan \theta}\\ \\\sin(\frac{\pi}{2}+\theta)&=&\cos\theta \\ \cos(\frac{\pi}{2}+\theta)&=&-\sin\theta \\ \tan(\frac{\pi}{2}+\theta)&=&-\frac{1}{\tan \theta} \end{eqnarray*}\]
補角
\[\begin{eqnarray*}\sin(\pi-\theta)&=&\sin\theta \\ \cos(\pi-\theta)&=&-\cos\theta \\ \tan(\pi-\theta)&=&-\tan \theta\\ \\\sin(\pi+\theta)&=&-\sin\theta \\ \cos(\pi+\theta)&=&-\cos\theta \\ \tan(\pi+\theta)&=&\tan \theta \end{eqnarray*}\]

コメント